Don't you know how to draw linear equations without using a calculator? Fortunately, drawing a graph of a linear equation is fairly easy if you know how. All you have to do is understand a few things about your equation and you will be able to do it. Let's start.
Step
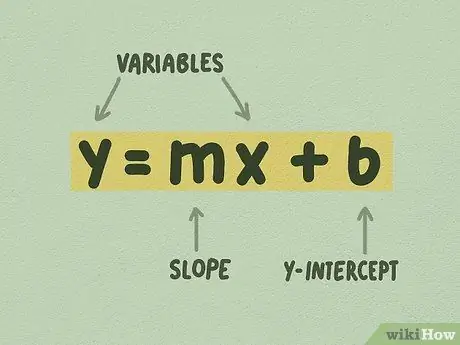
Step 1. Make sure the linear equation has the form y = mx + b
This shape is called the y-intercept form, and is probably the easiest form to use to graph linear equations. The value in the equation does not have to be an integer. Often, you'll see an equation that looks like this: y = 1/4x + 5, where 1/4 is m and 5 is b.
- m is called the "slope", or sometimes the "gradient". The slope is defined as the increase over the side, or the change in y divided by the change in x.
- b is defined as "y-intercept". The y-intercept is the point where the line intersects the Y-axis.
- x and y are variables. You can solve for a specific x value, for example, if you have a point y and know the values of m and b. However, x, never has only one value: its value changes as the line goes up or down.
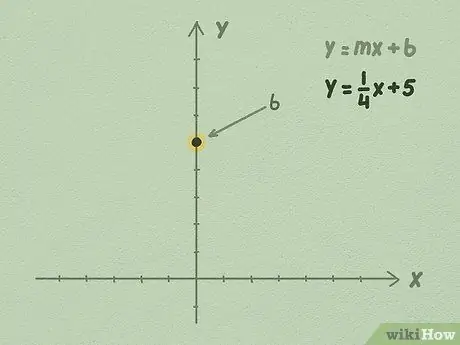
Step 2. Draw the number b on the Y axis
Your b value will always be a rational number. Whatever the number b is, find its value on the Y axis, and place the number at a point on the vertical axis.
For example, let's use the equation y = 1/4x + 5. Since the last number is b, we know that b is equal to 5. Move up 5 points on the Y axis and mark the points. This is where your straight line will intersect the Y axis
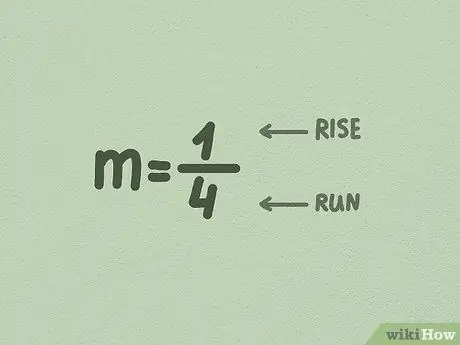
Step 3. Convert m to a fraction
Often, the number in front of x is already a fraction, so you don't need to change it. But if not, just change it by placing the value of m under the number 1.
- The first number (the numerator) is the increment in ascending divided by the side. This number indicates how far the line goes up, or vertically.
- The second number (the denominator) is the side in ascending divided by the side. This number indicates how far the line moves sideways, or horizontally.
- For example:
- The slope of 4/1 moves 4 points up for every 1 point to the side.
- The slope of -2/1 moves 2 points down for every 1 point to the side.
- A slope of 1/5 moves 1 point up for every 5 points to the side.
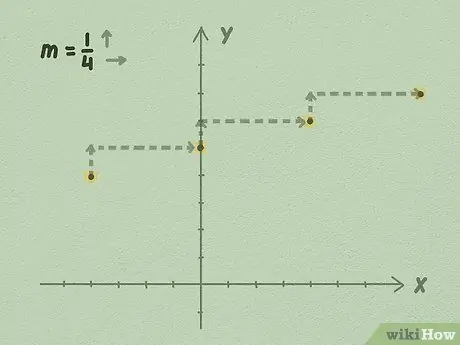
Step 4. Start extending the line from b using the slope, or up divided by the side
Start with your b value: we know that the equation goes past this point. Extend the line by taking your slope and using its value to get the points in the equation.
- For example, using the illustration above, you can see that for every 1 point up, the line moves 4 points to the right. This happens because the slope of the line is 1/4. You extend the line indefinitely to both sides, continuing to use up divided by the side to graph the line.
- Slope is positive when moving up, while slope is negative when moving down. A slope of -1/4 for example, will move down 1 point for every 4 points sideways.
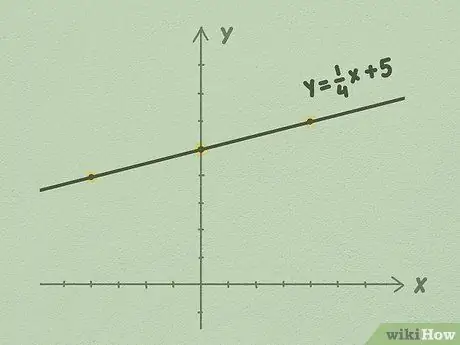
Step 5. Continue extending the line, using a ruler and making sure to use the slope, m, as a guide
Extend the line indefinitely and you're done graphing your linear equation. Pretty easy, right?






