Radians and degrees are two units used to measure angles. As you already know, a circle is made up of 2π radians, which is the equivalent of 360°; these two values represent the "one time circumference" of the circle. Thus, 1π radians represents a circle's circumference of 180°, making 180/π a perfect conversion tool for converting radians to degrees. To convert radians to degrees, you just need to multiply the radian value by 180/π. If you want to know how to do it and understand the concepts in the process, see Step 1 to get started.
Step

Step 1. Know that radians is equal to 180 degrees
Before you start the conversion process, you should know that radians = 180°, which is equivalent to half the circumference of a circle. This is important because you will be using 180/π as the conversion metric. This is because 1π radians is equal to 180/π degrees.
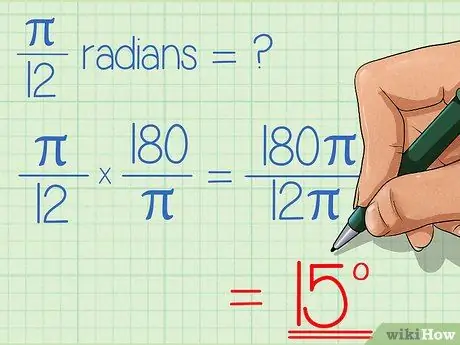
Step 2. Multiply radians by 180/π to convert them to degrees
As simple as that. Let's say you're working with /12 radians. Then, you have to multiply it by 180/π and simplify if needed. Here's how you do it:
- /12 x 180/π =
- 180π/12π 12π/12π =
- 15°
- /12 radians = 15°

Step 3. Practice with some examples
If you really want to understand the process, try converting from radians to degrees with a few additional examples. Here are some other examples you can work with:
- Example 1: 1/3π radians = /3 x 180/π = 180π/3π 3π/3π = 60°
- Example 2: 7/4π radians = 7π/4 x 180/π = 1260π/4π 4π/4π = 315°
- Example 3: 1/2π radians = /2 x 180/π = 180π /2π 2π/2π = 90°

Step 4. Remember that there is a difference between "radian" and "π radian"
If you say 2π radians or 2 radians, then you are not using the same term. As you know, 2π radians equal 360 degrees. However, if you are working with 2 radians, then if you want to convert it to degrees, you have to calculate 2 x 180/π. You'd get 360/π or 114, 5. This is a different answer because, if you don't work with radians, then doesn't cancel each other out in the equation and the results are different values.
Tips
- When multiplying, leave pi in your radians as a symbol and not its decimal approximation, so that it's easier for you to omit it in your calculations.
- Many graphing calculators have a function to convert units or you can download a program to convert units. Ask your math teacher if such a function is included in your calculator.






