Dividing two-digit numbers is very similar to dividing single-digit numbers, but is a bit longer and takes practice. Since most of us don't memorize the 47 times table, we need to go through the division process; however, there are tricks you can learn to speed things up. You will also become more fluent with practice. Don't be discouraged if you feel a bit sluggish at first.
Step
Part 1 of 2: Dividing by a Two-Digit Number

Step 1. Look at the first digit of the larger number
Write the problem as long division division. As with simple division, you can start by looking at the smaller number, and asking "Can the number fit into the first digit of the larger number?"
Say the problem is 3472 15. Ask "Can 15 get into 3?" Since 15 is clearly greater than 3, the answer is "no", and we can move on to the next step

Step 2. Look at the first two digits
Since a two-digit number cannot fit into a single-digit number, we will look at the first two digits of the numerator, just as in ordinary division problems. If you still have the impossible division problem, look to the first three digits of the number, but we don't need it in this example:
Can 15 get into 34? Yes, so we can start calculating the answer. (The first number doesn't have to fit perfectly, and just needs to be smaller than the second number.)
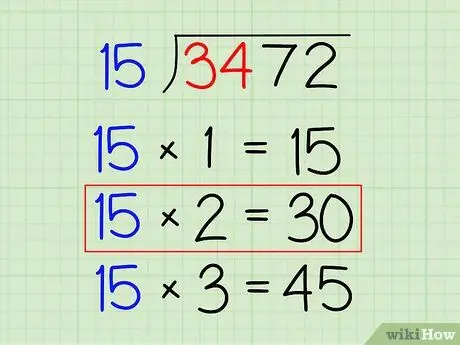
Step 3. Guess a little
Find out exactly how much the first number can fit into the other numbers. You may already know the answer, but if you don't, take a guess and check your answer through multiplication.
-
We need to solve 34 15, or "how many 15 can fit into 34"? You are looking for a number that can be multiplied by 15 to get a number that is less than but very close to 34:
- Can 1 be used? 15 x 1 = 15, which is smaller than 34, but keep guessing.
- Can 2 be used? 15 x 2 = 30. This answer is still smaller than 34 so 2 is a better answer than 1.
- Can 3 be used? 15 x 3 = 45, which is greater than 34. This number is too high so the answer is definitely 2.
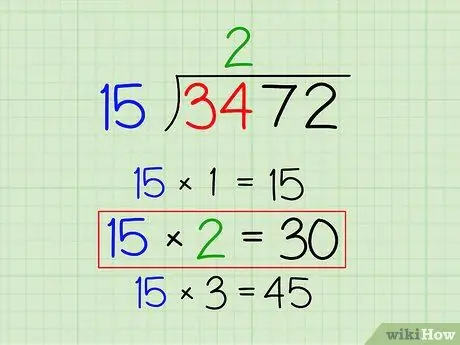
Step 4. Write the answer above the last digit used
If you're working on this problem as long division division, you should be familiar with this step.
Since you're counting 34 15, write your answer, 2, in the answer line above the number "4."
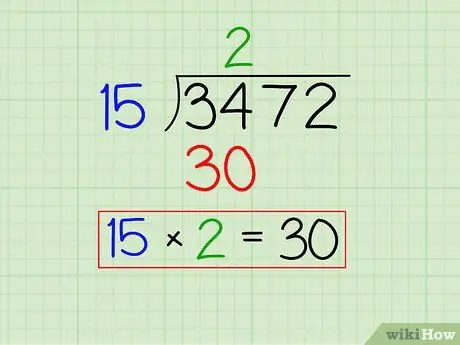
Step 5. Multiply the answer by the smaller number
This step is the same as in regular long-order division, except that we use a two-digit number.
Your answer is 2 and the smaller number in the problem is 15 so we calculate 2 x 15 = 30. Write "30" under "34"
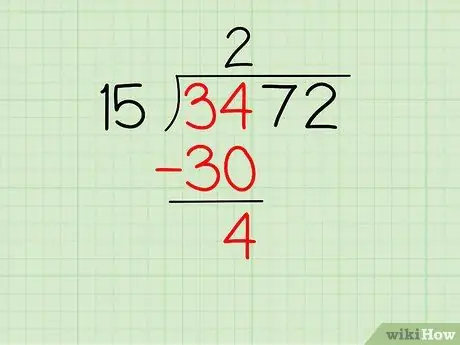
Step 6. Subtract both numbers
The result of the previous multiplication is written under the larger starting number (or part thereof). Do this part as a subtraction operation and write the answer on the line below it.
Solve 34 - 30 and write the answer on a new line below it. The answer is 4, which is the "remainder" after 15 is entered into 34 twice and we need it in the next step

Step 7. Lower the next digit
Like a regular division problem, we will continue to work on the next digit of the answer until it is finished.
Leave the number 4 where it is, and subtract "7" from "3472" so you now have 47

Step 8. Solve the next division problem
To get the next digit, simply repeat the same steps as above to apply to this new problem. You can go back to guessing to find the answer:
-
We need to solve 47 15:
- The number 47 is bigger than our last number so the answer will be higher. Let's try four: 15 x 4 = 60. Wrong, the answer is too high!
- Now, let's try three: 15 x 3 = 45. This result is smaller and very close to 47. Perfect.
- The answer is 3 and we write it above the number "7" in the answer line.
- If you get a problem like 13 15, where the numerator is smaller than the denominator, drop down the third digit before solving it.

Step 9. Continue using long division
Repeat the long division steps used earlier to multiply the answer by the smaller number, then write the result under the larger number, then subtract to find the next remainder.
- Remember, we just calculated 47 15 = 3, and now want to find the remainder:
- 3 x 15 = 45 so write "45" under 47.
- Solve 47 - 45 = 2. Write "2" under 45.

Step 10. Find the last digit
As before, we bring the next digit from the original problem so that we can solve the next division problem. Repeat the above steps until you find each digit in the answer.
- We get 2 15 as the next problem, which makes no sense.
- Decrease one digit so that you now get 22 15.
- 15 can go to 22 once so write "1" at the end of the answer line.
- Our answer now is 231.

Step 11. Find the remainder
Do one last subtraction to find the final remainder, and we're done. In fact, if the answer to the subtraction problem is 0, you don't even have to write down the remainder.
- 1 x 15 = 15 so write 15 under 22.
- Count 22 - 15 = 7.
- We no longer have digits to go down so simply write "remaining 7" or "S7" at the end of the answer.
- The final answer is: 3472 15 = 231 remaining 7
Part 2 of 2: Guessing Well

Step 1. Round to the nearest ten
Sometimes, the number of two-digit numbers that can fit into a larger number can't be easily seen. One trick to make it easier is to round a number to the nearest ten. This method is good for smaller division problems, or some long division problems.
For example, let's say we are working on problem 143 27, but have a hard time guessing the number of 27 that can fit into 143. For now, assume the problem is 143 30

Step 2. Count the smaller numbers with your fingers
In our example, we could count 30 instead of 27. Counting 30 is easier once you get used to it: 30, 60, 90, 120, 150.
- If you're still having trouble, just count multiples of 3 and put a 0 at the end
- Count until you get a result greater than the large number in problem (143), then stop.

Step 3. Find the two most likely answers
We didn't exactly reach 143, but there are two numbers that do come close: 120 and 150. Let's see how many fingers count to get it:
- 30 (one finger), 60 (two fingers), 90 (three fingers), 120 (four fingers). So, 30 x four = 120.
- 150 (five fingers) up to 30 x five = 150.
- 4 and 5 are the most likely answers to our questions.

Step 4. Test both numbers with the original problem
Now that we have two guesses, let's get to the original problem, which is 143 27:
- 27 x 4 = 108
- 27 x 5 = 135

Step 5. Make sure the numbers can't get any closer
Since both numbers are close and less than 143, let's try to bring it closer with multiplication:
- 27 x 6 = 162. This number is greater than 143 so it cannot be the correct answer.
-
27 x 5 is the closest without exceeding 143 so 143 27 =
Step 5. (plus remaining 8 because 143 - 135 = 8.)
Tips
If you don't like multiplying by hand when doing long division, try dividing the problem into multiple digits and solving each section in your head. For example, 14 x 16 = (14 x 10) + (14 x 6). Write down 14 x 10 = 140 so you don't forget. Then, calculate: 14 x 6 = (10 x 6) + (4 x 6). The results are 10 x 6 = 60 and 4 x 6 = 24. Add up 140 + 60 + 24 = 224 and you get the final answer
Warning
- If, at any one time, subtraction yields a number negative, your guess is too big. Eliminate all steps and try to guess the smaller number.
- If, at some point, the subtraction results in a number greater than the denominator, your guess is not big enough. Eliminate all steps and try to guess the bigger number.






