To multiply fractions, all you have to do is multiply the numerator and denominator and simplify the result. To divide fractions, all you have to do is reverse the numerator and denominator of one fraction, multiply the result by another, and simplify. If you want to know how to quickly divide and multiply fractions, follow these steps.
Step
Method 1 of 2: Multiply Fractions
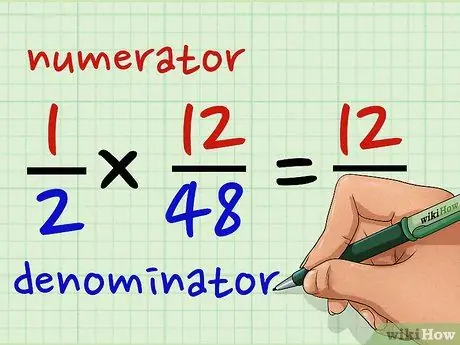
Step 1. Multiply the numerator of the fraction
The numerator is the top number of the fraction and the denominator is the bottom number of the fraction. The first step in multiplying fractions is to align the fractions so that the numerator and denominator are next to each other. If you're multiplying the fraction 1/2 by 12/48, the first thing you should do is multiply the numerators, 1 and 12. 1 x 12 = 12. Write down the product, 12, in the numerator section of your answer.
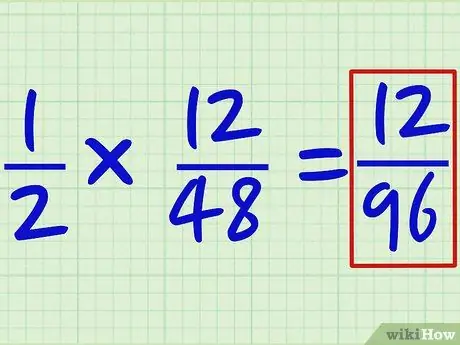
Step 2. Multiply the denominator of the fraction
Now, you just need to do the same as the numerator. Multiply 2 and 48 to find the new denominator. 2 x 48 = 96. Write this answer in the denominator of your answer. Thus, the new fraction is 12/96.
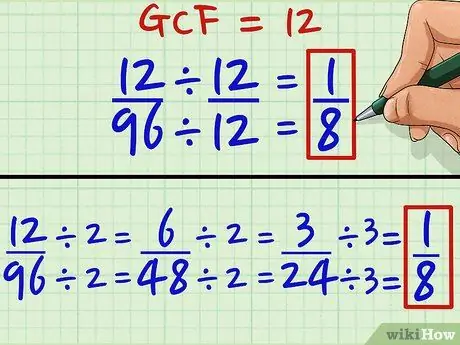
Step 3. Simplify the fraction
The final step is to simplify the result if you can. To simplify a fraction, you have to find the greatest common factor (GCF) of the numerator and denominator. The GCF is the largest number that can divide the two numbers. In problems 12 and 96, it happens that 12 can divide 96. So, divide 12 by 12 to get 1, and divide 96 by 12 to get 8. Thus, 12/96 12/12 = 1/8.
If both numbers are even numbers, you can also start dividing by 2 and continue dividing by 2. 12/96 2/2 = 6/48 2/2 = 3/24. Then, you'll notice that 24 is divisible by 3, so you can divide the numerator and denominator by 3 to get 1/8. 3/24 3/3 = 1/8
Method 2 of 2: Divide Fractions

Step 1. Reverse the denominator and numerator of one of the fractions and convert the division sign to multiplication
For example, you divide 1/2 by 18/20. Now, flip the numerator from 18/20 to 20/18 and convert the division sign to multiplication. So, 1/2 18/20 = 1/2 x 20/18.
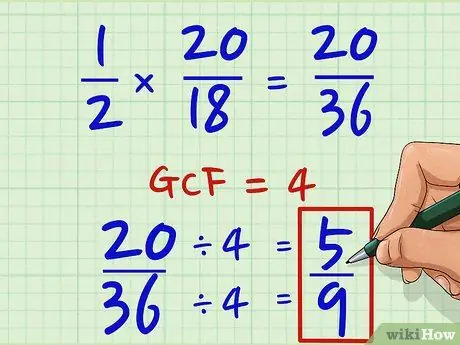
Step 2. Multiply the numerator and denominator of the fraction and simplify your answer
Now, do the same as multiplying fractions. If you multiply the numerators, 1 and 20, the result is 20 in the numerator. If you multiply the denominators, 2 and 18, you get 36 in the denominator. The result of multiplying fractions is 20/36. 4 is the largest number that can divide the numerator and denominator of this fraction, so divide both by 4 to simplify the answer. 20/36 4/4 = 5/9.
Tips
- Double check your work.
- Remember: whole numbers can be written as a fraction: 2 equals 2/1.
- Never forget to simplify.
- Cross-doodle can be used at any time to save time while simplifying. Crossing out is dividing diagonally by the same factor. For example, the question (8/20)*(6/12) can be crossed out to be (2/10)*(3/3).
Warning
- Do it step by step. This will reduce the possibility of errors occurring.
- Remember to simplify fractions to their simplest form. Simplification that is not simple is the same as not simplifying.
- There is always more than one way to get things done in math. However, just because your answer is correct when you solve a problem in a different way, doesn't mean it's always the right way. Another way to divide fractions, for example, is cross product, which multiplies the diagonals.






