"Inductance" refers to mutual inductance, which is when an electric circuit creates a voltage due to changes in current in the other strand, and self inductance, which is the creation of a voltage in the strand due to its own current. In both forms, inductance is the ratio of voltage to current and is measured in a unit called the henry, which is defined as 1 volt second per ampere. Since the henry is such a large unit, inductance is usually measured in electenry (mH), which is one per thousand henry, or microhenry (uH), aka one per million henry. Follow the following methods to measure the inductance of an inductor.
Step
Method 1 of 3: Measuring Inductance on a Voltage Current Graph
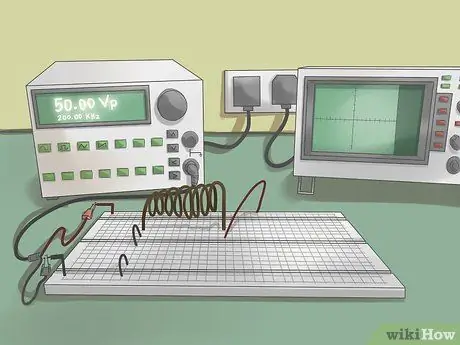
Step 1. Connect the inductor to a pulsed voltage source
Keep the pulse below 50%.
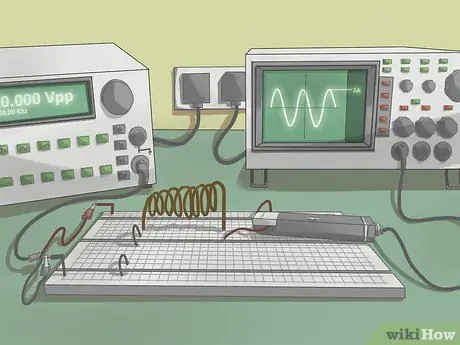
Step 2. Set up the flow monitor
You will need to connect a current sense resistor to the strand, or use a current probe (metal tip to measure). Both must be connected to the oscilloscope.
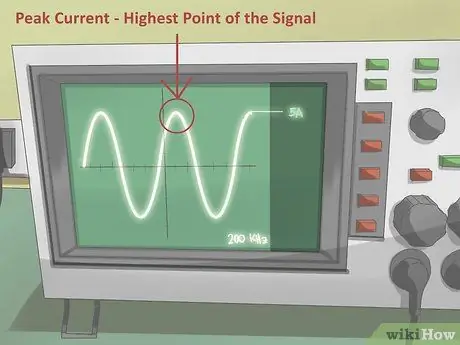
Step 3. Read the peak current and the amount of time between each voltage pulse
Peak current will be measured in amperes, and time between pulses will be measured in microseconds.
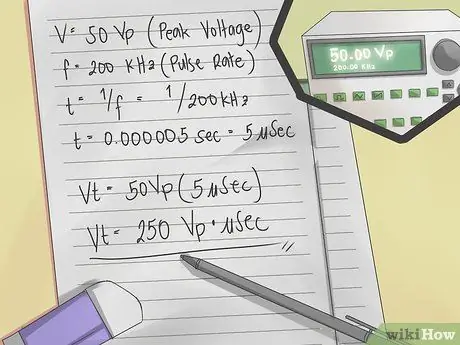
Step 4. Multiply the voltage delivered in each beat by the length of each beat
For example, if 50 volts is applied every 5 microseconds, the calculation is 50 x 5 = 250 volt-microseconds.
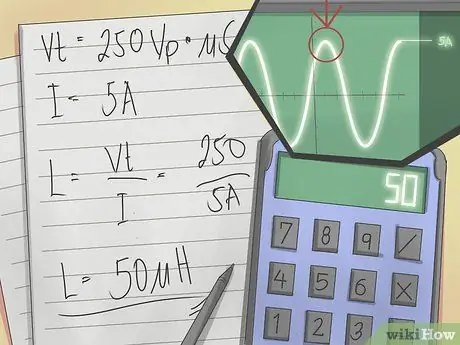
Step 5. Divide by peak current
Continuing the example above, we will divide the product of the voltage and pulse length by the peak current. If the peak current is 5 amperes, the inductance obtained is 250 volt-microseconds / 5 amperes = 50 microhenry.
Although the calculations are simple, the preparation for this method of searching for induction is more complicated than for other methods
Method 2 of 3: Measuring Inductance Using Resistors
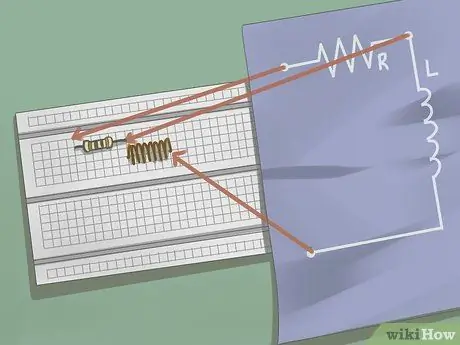
Step 1. Connect an inductor with a resistor of known resistance to form a series circuit
The resistor must be within 1% or less. The series circuit forces current through the resistor and inductor under test. One of the terminals of the resistor and inductor must touch each other.
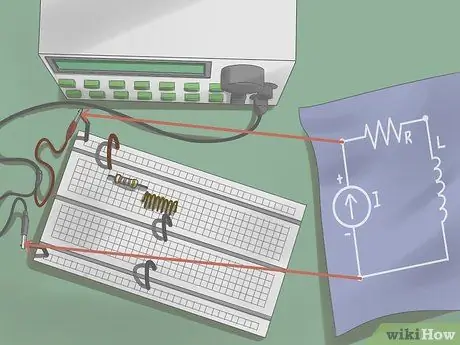
Step 2. Run current through the strand
This is done with a function generator. The function generator stimulates the current that the inductor and resistor will receive when used.
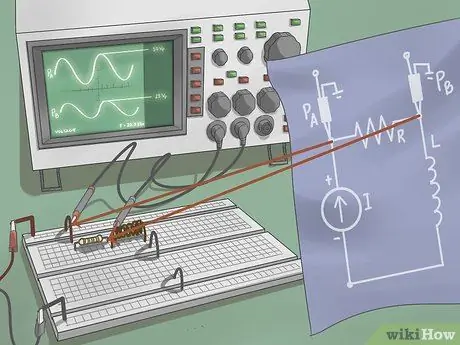
Step 3. Monitor the input voltage and the voltage where the inductor and resistor meet
Adjust the frequency until the combined voltage at the junction of the inductor and resistor is half the input voltage.
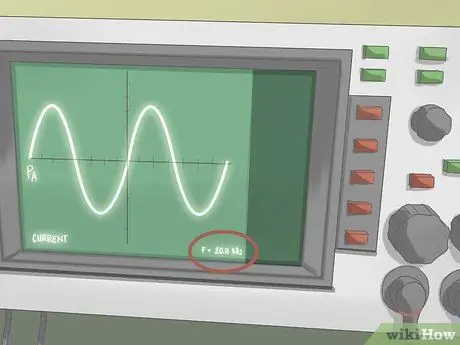
Step 4. Find the current frequency
The frequency of the current is calculated in kilohertz.

Step 5. Calculate the inductance
In contrast to the voltage and current method, the preparation of this test is easier, but the calculations will be more complicated. The details are as follows:
- Multiply the resistance of the resistor by the cubic root. If the resistor has a resistance of 100 ohms, multiply it by 1.73 (cubic root value to two decimal places) to get 173
- Divide the result of the calculation above by the result of 2 pi times the frequency. If the frequency is 20 kilohertz, the calculation is 2 x 3.14 (pi to two decimal places) x 20 = 125. 6. To get the inductance, divide 173 by 125.6 to get 1.38 millihenry
- mH = (R x 1.73) / (6.28 x (Hz / 1,000))
- Example: it is known that R = 100 and Hz = 20,000
- mH = (100 X 1.73) / (6.28 x (20,000 / 1,000)
- mH = 173 / (6.28 x 20)
- mH = 173 / 125, 6
- mH = 1.38
Method 3 of 3: Measuring Inductance Using Capacitors and Resistors
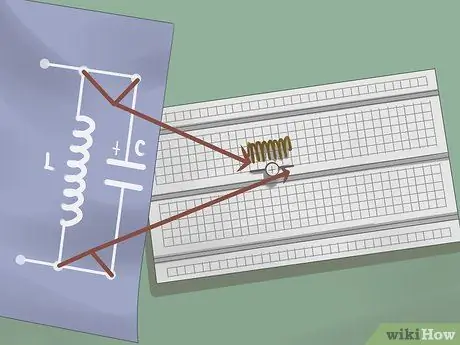
Step 1. Connect the inductor parallel to the capacitor of known capacitance
An inductor connected in parallel with a capacitor will produce a parallel circuit. Use capacitors with a tolerance of 10% or less.
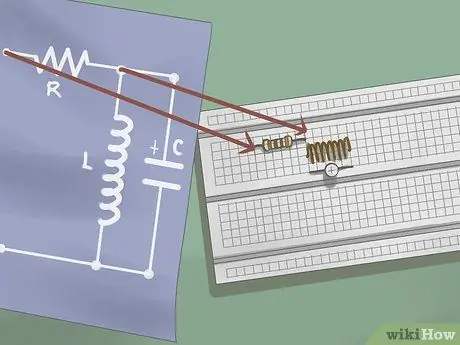
Step 2. Connect the circuit in parallel with in series with the resistor
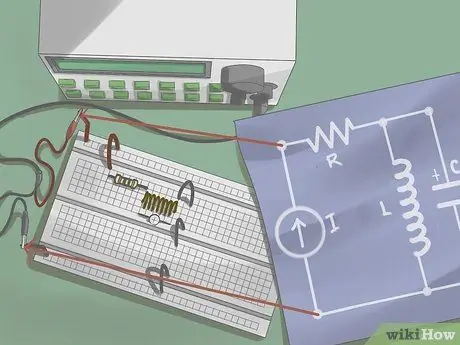
Step 3. Flow current through the circuit
Again, use the function generator.
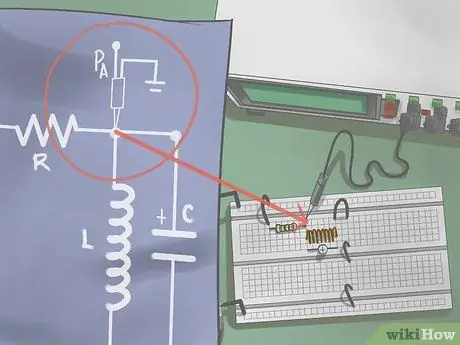
Step 4. Place the probe from the oscilloscope along the parallel circuit
Step 5. Change the frequency of the function generator from lowest to highest

Step 6. When changing the frequency, look at the resonance frequency of the strand, where the oscilloscope produces the highest waveform
Step 7. Calculate the inductance L = 1/((2 pi f)^2 * C)
The resonant frequency of the LC strand is measured in Hertz, and you already know the frequency f = 1/ (2 pi sqrt(L*C)). For example, if the value of the resonant frequency is 5000Hz, and the capacitance is 1 uF (1.0e-6 farads), the inductance is 0.001 henry, or 1000 uH.
Tips
- When a group of inductors are connected in series, the total inductance is the sum of the inductances of each inductor. When a group of inductors are connected in parallel to form a parallel circuit, one inductance per total is the sum of each one per inductance of each inductor in the strand.
- Inductors can be arranged as rod coils, ring-shaped cores, or from thin films. The more windings in an inductor, or the greater the cross-sectional area, the greater the inductance. Long inductors have weaker inductance than short inductors.
Warning
- Inductance can be measured directly with an inductance meter, but these meters are hard to find. Most inductance meters are made only to measure low currents.
- Sorry, the calculation in Method 2 Step 5 is wrong. You should be dividing by the square of 3, not multiplying. So, the correct formula is L = R / (sqrt(3)*2*pi*f)






