The buoyant force is the force opposite to gravity, which affects all objects submerged in a fluid. When an object is placed in a fluid, the object's mass presses against the fluid (liquid or gas), while the buoyant force pushes the object against gravity. In general terms, this buoyant force can be calculated by the equation Fa = Vt × × g, with Fa is the buoyant force, Vt is the volume of the submerged object, is the density of the fluid, and g is the gravitational force. To learn how to determine the buoyancy of an object, see Step 1 below to get started.
Step
Method 1 of 2: Using the Equation of Buoyancy
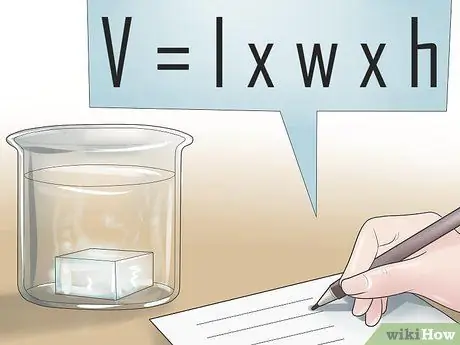
Step 1. Find the volume of the submerged part of the object
The buoyant force acting on an object is proportional to the volume of the submerged object. In other words, the larger the submerged solid part of the object, the greater the buoyant force acting on the object. This means that objects that are submerged in a liquid, have a buoyant force that pushes the object up. To begin calculating the buoyant force acting on an object, your first step is usually to determine the volume of the object submerged in the fluid. For the equation of buoyancy, this value should be in meters3.
- For an object completely submerged in a fluid, the volume submerged is equal to the volume of the object itself. For objects that float above the surface of the fluid, only the volume below the surface is calculated.
- For example, suppose we want to find the buoyant force acting on a rubber ball floating on water. If the rubber ball is a perfect sphere with a diameter of 1 m and floats with half of it submerged under water, we can find the volume of the submerged portion by finding the total volume of the sphere and dividing by two. Since the volume of the sphere is (4/3) (radius)3, we know that the volume of our sphere is (4/3)π(0, 5)3 = 0.524 meters3. 0, 524/2 = 0.262 meters3 sink.

Step 2. Find the density of your fluid
The next step in the process of finding buoyancy is to define the density (in kilograms/meter3) of the fluid in which the object is immersed. Density is a measurement of the mass of an object or substance relative to its volume. If given two objects with the same volume, the object with the greater density will have more mass. According to the rule, the greater the density of the fluid in which the object is submerged, the greater the buoyant force. With fluids, usually the easiest way to determine density is simply to look it up in a reference material.
- In our example, our ball floats in water. By looking into academic sources, we can find that water has a density of approx. 1,000 kilograms/meter3.
- Other widely used fluid densities are listed in engineering sources. One of the lists can be found here.
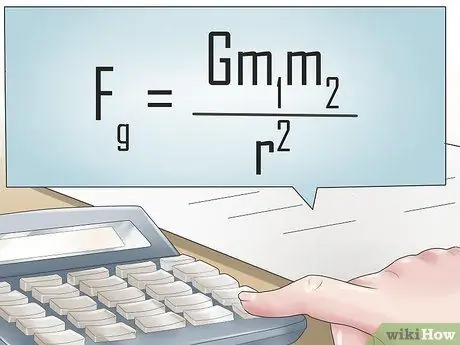
Step 3. Find the force of gravity (or some other downward force)
Whether an object sinks or floats in a fluid, it always has a gravitational force. In the real world, the downward force constant is equal to 9.81 newtons/kilogram. However, in situations where other forces, such as the centrifugal force, act on the fluid and the object submerged in it, this force must also be taken into account to determine the net downward force for the entire system.
- In our example, we are working with an ordinary, static system, so we can assume that the only downward force acting on fluids and objects is the general gravitational force - 9.81 newtons/kilogram.
- However, what if our ball, which is floating in a bucket of water, is swung in a circle in a horizontal direction at high speed? In this case, assuming the bucket is being swung fast enough that the water and the ball don't spill, the downward force in this situation will be derived from the centrifugal force created by the swing of the bucket, not from Earth's gravity.

Step 4. Multiply volume × density × gravity
If you have the volume value of your object (in meters3), the density of your fluid (in kilograms/meter3), and the force of gravity (the downward force on your system), so finding buoyancy is very easy. Just multiply these three values to find the buoyant force in newtons.
Let's solve our example problem by plugging our values into the equation Fa = Vt × × g. Fa = 0.262 meters3 × 1,000 kilograms/meter3 × 9.81 newtons/kilogram = 2,570 newtons.

Step 5. See if your object floats by comparing the buoyancy to the gravitational force
Using the buoyancy equation, it's easy to find the force that pushes an object up and out of the fluid. However, with a little extra effort, it is also possible to determine if an object will float or sink. Just find the buoyant force for the whole object (in other words, use the entire volume for the value of Vt), then find the gravitational force pushing it down with the equation G = (mass of the object)(9.81 meters/second2). If the buoyant force is greater than the gravitational force, the object will float. On the other hand, if the gravitational force is greater than the buoyant force, the object will sink. If the magnitudes are the same, the object is said to float.
-
For example, say we want to know if a wooden cylindrical barrel with a mass of 20 kilograms and a diameter of 0.75 m and a height of 1.25 m will float in water. This problem will use several steps:
- We can find the volume with the formula for the volume of the cylinder V = (radius)2(tall). V = (0, 375)2(1, 25) = 0.55 meters3.
- Next, assuming that the magnitude of gravity is ordinary and that of water of ordinary density, we can find the buoyant force of the barrel. 0.55 meters3 × 1000 kilograms/meter3 × 9.81 newtons/kilogram = 5,395, 5 newtons.
- Now, we need to find the gravitational force of the barrel. G = (20 kg)(9.81 meters/second2) = 196.2 newtons. This force is less than the buoyant force, so the barrel will float.

Step 6. Use the same approach if your fluid is a gas
When working on buoyancy problems, don't forget that the fluid in which the object is submerged does not have to be a liquid. Gases are also fluids, and, although gases have a very low density compared to other substances, they can still support certain masses of objects floating in the gas. A simple helium balloon is proof of that. Because the gas in the balloon is less dense than the surrounding fluid (ambient air), the balloon floats!
Method 2 of 2: Performing a Simple Buoyancy Experiment

Step 1. Place a small bowl or cup inside a larger bowl
With some household items, it's easy to see the principles of buoyancy in experiment! In this simple experiment, we will demonstrate that a submerged object experiences a buoyant force because it displaces a volume of fluid equal to the volume of the submerged object. As we do this, we will also demonstrate a practical way to find the buoyant force of an object with this experiment. To start, place a small, open container, such as a bowl or cup, inside a larger container, such as a large bowl or bucket.

Step 2. Fill the small container to the brim
Next, fill the smaller inner container with water. You want the water to be as high as the container without spilling. Be careful here! If you spill water, empty the larger container before trying again.
- For the purposes of this experiment, it is okay to assume that water has a general density of 1000 kilograms/meter3. Unless you're using seawater or a completely different liquid, most types of water have nearly the same density as this reference value so a small difference won't change our results.
- If you have eye drops, this can be very useful for raising the water level in a small container.

Step 3. Submerge the small object
Next, look for a small object that will fit into a small container and won't be damaged by water. Find the mass of this object in kilograms (you may want to use a scale or balance that can take grams and convert them to kilograms). Then, without getting your fingers wet, slowly but surely, dip the object in the water until it starts to float or you can hold it slightly and then release it. You will notice that some of the water in the small container will spill into the outer container.
For the purposes of our example, suppose we dip a toy car with a mass of 0.05 kilograms into a small container. We don't need to know the volume of this car to calculate its buoyancy because we'll see that in the next step

Step 4. Collect and count the spilled water
When you submerge an object in water, it displaces some of the water - otherwise there will be no place to put the object in the water. When an object pushes the water out, the water pushes back, creating a buoyant force. Take the spilled water from a small container and pour it into a small measuring cup. The volume of water in the measuring cup is equal to the volume of the object submerged.
In other words, if your object floats, the volume of water that spills out will be equal to the volume of the object that is submerged under the surface of the water. If your object sinks, the volume of water that spills out is equal to the total volume of the object

Step 5. Calculate the mass of the spilled water
Since you know the density of water and you can measure the volume of water that spills in the measuring cup, you can find its mass. Just change the volume to meters3 (online conversion aids, like this one, can help) and multiply by the density of water (1,000 kilograms/meter3).
In our example, suppose our toy car sinks into a small container and moves about two tablespoons (0.0003 meters3). To find the mass of our water, we will multiply it by its density: 1,000 kilograms/meter3 × 0.0003 meters3 = 0.03 kilograms.

Step 6. Compare the mass of the spilled water with the mass of the object
Now that you know the mass of the object you are submerging in the water and the mass of the water that has spilled, compare them to see which mass is larger. If the mass of an object submerged in a small container is greater than the spilled water, the object will sink. On the other hand, if the mass of the spilled water is greater, the object will float. This is the principle of buoyancy in experiment - in order for an object to float, it must displace an amount of water with a mass greater than the mass of the object itself.
- Thus, objects with low mass but large volume are the types of objects that float most easily. This property means that hollow objects float very easily. Imagine a canoe - the canoe floats well because it is hollow inside, so it can move a lot of water without having to have a large mass. If the canoe is not hollow (solid), then the canoe will not float properly.
- In our example, the car has a greater mass (0.05 kilograms) than the spilled water (0.03 kilograms). This agrees with what we observe: the cars sink.






