Adding fractions is a very useful knowledge. This skill is very easy to learn and use when working on math problems from elementary to high school. This article explains how to add fractions so you can do it in just a few minutes.
Step
Method 1 of 2: Adding Fractions with the Same Denominator

Step 1. Check the denominator (the number under the bisector) of each fraction
If the numbers are the same, then you're adding fractions with the same denominator. If the denominators are different, read the second method.

Step 2. Answer the following 2 questions
By reading the last step in this method, you should be able to add up the fractions of the following two questions.
- Problem 1: 1/4 + 2/4
- Problem 2: 3/8 + 2/8 + 4/8
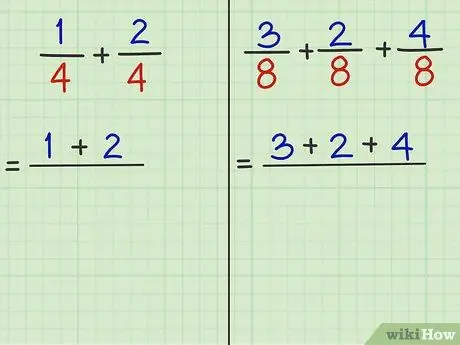
Step 3. Collect the numerators (the numbers above the divide) and add them up
The numerator is the number above the quotient. No matter how many fractions you want to add, you can add the numerators right away if the denominators are the same.
- Problem 1: 1/4 + 2/4 is the fraction to be added. "1" and "2" are numerators. So, 1 + 2 = 3.
- Problem 2: 3/8 + 2/8 + 4/8 is the fraction to be added. "3" and "2" and "4" are numerators. So, 3 + 2 + 4 = 9.
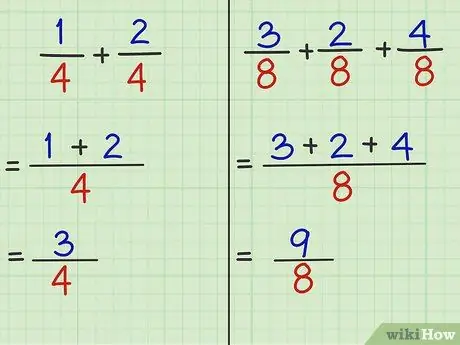
Step 4. Determine the new fraction from the sum
Write down the numerator obtained in step 2. This number is new numerator. Write the denominator, which is the same number under the bisector of each fraction. You don't need to do the calculations if the denominators are the same. This figure is new denominator and always equals the old denominator when you add fractions with the same denominator.
- Problem 1: 3 is the new numerator and 4 is the new denominator. Thus, the answer to question 1 is 3/4. 1/4 + 2/4 = 3/4.
- Problem 2: 9 is the new numerator and 8 is the new denominator. Thus, the answer to question 2 is 9/8. 3/8 + 2/8 + 4/8 = 9/8.
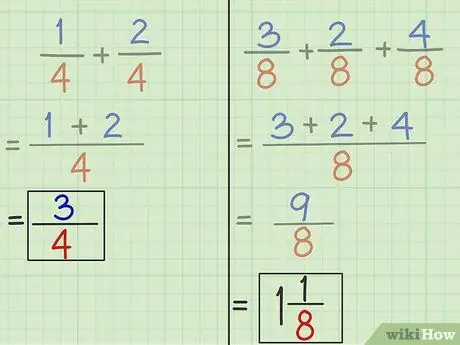
Step 5. Simplify fractions if needed
Don't forget to simplify the new fraction so that the writing is simpler.
-
If the numerator bigger instead of a denominator like the result of the addition of problem 2, this means we get 1 whole month after simplifying the fraction. Divide the numerator by the denominator or 9 divided by 8. The result is an integer 1 remaining 1. Write integers in front of the fraction and the remainder becomes the numerator of a new fraction with the same denominator.
9/8 = 1 1/8.
Method 2 of 2: Adding Fractions with Different Denominators

Step 1. Check the denominator (the number under the quotient) of each fraction
If the denominators are different, you're add fractions with different denominators. Read the following steps because you have to make the denominators equal before adding fractions.
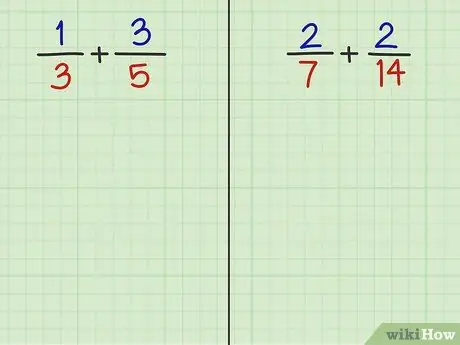
Step 2. Solve the following 2 questions
By reading the last step in this method, you should be able to add up the fractions of the following two questions.
- Problem 3: 1/3 + 3/5
- Question 4: 2/7 + 2/14
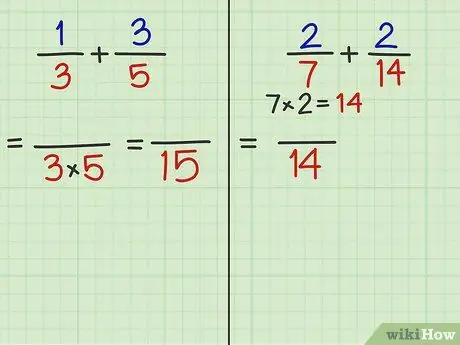
Step 3. Match the denominators
To do this, multiply the denominators of the two fractions above. An easy way to equalize the denominators is to multiply the denominators of the two fractions. If one of the denominators is a multiple of the other, find the least common multiple of the two denominators.
-
Problem 3:
3 x 5 = 15. So, the new denominator of both fractions is 15.
-
Problem 4:
14 is a multiple of 7. Therefore, we only need to multiply 7 by 2 to get 14. Thus, the new denominator of both fractions is 14.
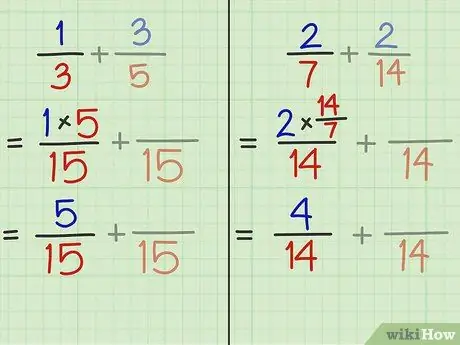
Step 4. Multiply the numerator and denominator of the first fraction by the denominator of the second fraction
This step doesn't change the value of the fraction, but the fraction looks to change to match the denominator. The fractional value remains the same.
-
Problem 3:
1/3 x 5/5 = 5/15.
-
Problem 4:
For this problem, we just need to multiply the first fraction by 2/2 to make the denominator the same.
2/7 x 2/2 = 4/14
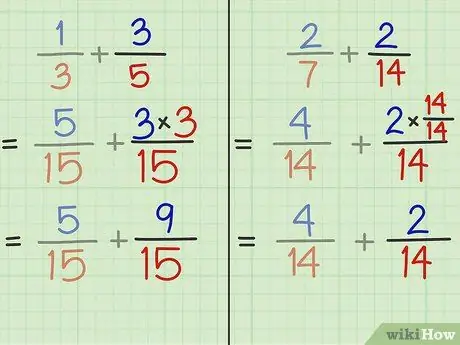
Step 5. Multiply the numerator and denominator of the second fraction by the denominator of the first fraction
Similar to the steps above, we don't change the value of the fraction, but the fraction looks to change to equalize the denominator. The fractional value remains the same.
-
Problem 3:
3/5 x 3/3 = 9/15.
-
Problem 4:
We don't need to multiply the second fraction because the denominators are the same.
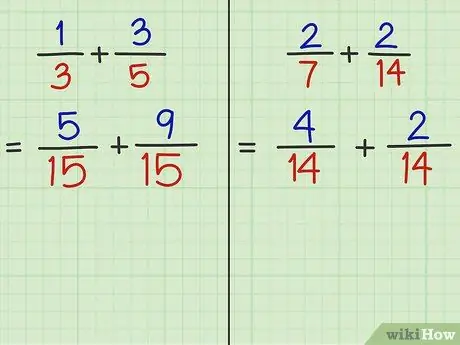
Step 6. Write the two new fractions in order
At this point, we haven't added the two fractions together, although we can. In the step above, we multiplied each fraction by 1. Now, we want to make sure the fractions we want to add have the same denominator.
-
Problem 3:
instead of 1/3 + 3/5, the fraction becomes 5/15 + 9/15
-
Problem 4:
Instead of 2/7 + 2/14, the fraction becomes 4/14 + 2/14
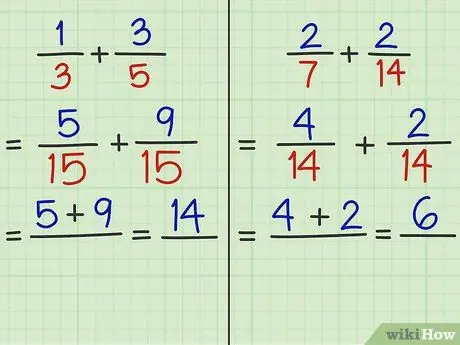
Step 7. Add the numerators of the two fractions together
The numerator is the number above the quotient.
-
Problem 3:
5 + 9 = 14. 14 is the new numerator.
-
Problem 4:
4 + 2 = 6. 6 is the new numerator.
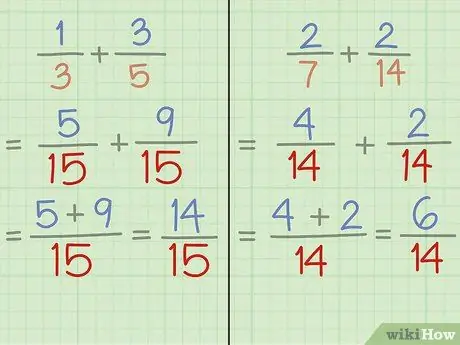
Step 8. Write the common denominator (in step 2) under the new numerator or use the denominator of the fraction multiplied by 1 to equalize the denominator
-
Problem 3:
15 is the new denominator.
-
Problem 4:
14 is the new denominator.

Step 9. Write a new numerator and a new denominator
-
Problem 3:
14/15 is the answer 1/3 + 3/5 = ?
-
Problem 4:
6/14 is the answer 2/7 + 2/14 = ?
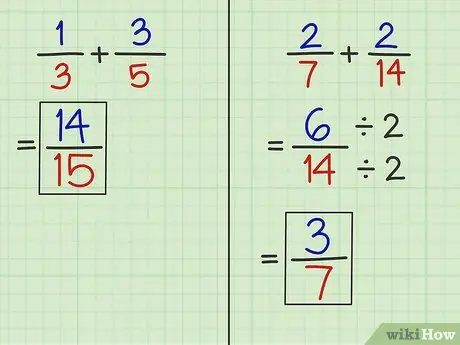
Step 10. Simplify and reduce fractions
To simplify fractions, divide the numerator and denominator by the greatest common factor of the two numbers.
-
Problem 3:
14/15 cannot be simplified.
-
Problem 4:
6/14 can be reduced to 3/7 after dividing the numerator and denominator by 2 as the greatest common factor of 6 and 14.
Tips
- Before adding fractions, make sure the denominators are the same.
- Don't add up the denominators. If the denominators are the same, use the number as the denominator after the fractions have been added.
- If you want to add fractions with numbers that consist of whole numbers and fractions, convert those numbers to fractions and add them up according to the instructions above.






