If you know how to calculate loan payments, you can plan your own budget so you won't be surprised later. It is recommended that you use an online loan calculator because calculating using a regular calculator that uses long formulas makes it easier for you to make mistakes.
Step
Method 1 of 3: Using an Online Calculator

Step 1. Open an online loan calculator
You can press the calculator in the samples section at the top of the page, then open it with Google Drive or download it so you can open it with Excel or another program. Additionally, open one of the following links:
- Bankrate.com and MLCalc are both simple calculators that also show you an entire table of your payment schedule, including your remaining debt.
- CalculatorSoup is especially useful for unusual payments or using incremented intervals. For example, mortgages in Canada are usually added every six months, or twice a year. (The calculator above assumes that interest is added monthly, and payments are made monthly.)
- You can create your own calculator in Excel, similar to the example from the wikiHow above.
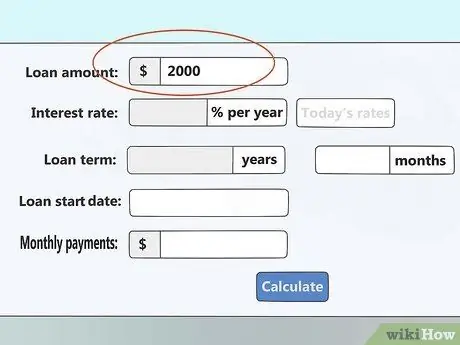
Step 2. Enter the loan amount
This is the total amount of money you borrowed. If you want to calculate a partially paid debt, enter the remaining debt you still owe.
This column might be entitled "base amount."
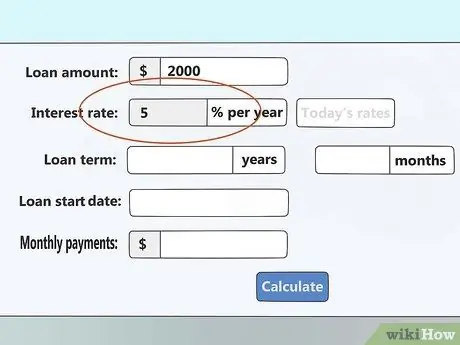
Step 3. Enter the interest rate
This is the current interest rate on your loan and is in percent. For example, if you have to pay a 6% interest rate, type a number
Step 6
The increment interval doesn't really matter here. The interest rate set should be the annual amount of interest, even if that interest is calculated more often

Step 4. Enter the loan term
This is the amount of time you plan to pay off your debt. Use the amount of time specified in the loan policy to calculate the minimum monthly payment that must be made. Use a shorter amount of time to calculate higher payments so you can pay off your debts quickly.
- Paying debts faster also means less money spent.
- Refer to the following table in this column to determine whether this calculator uses a monthly or annual system.
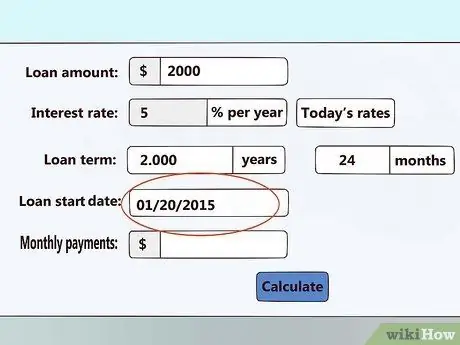
Step 5. Enter the start date
This is used to calculate on what date you will repay the loan.
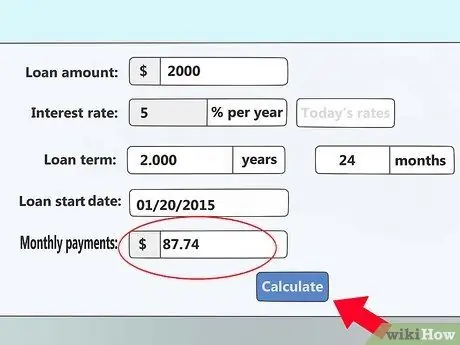
Step 6. Press calculate (calculate
) Some calculators will update the "Monthly Payment" field automatically after you enter the information. However, some have to wait for you to hit the "calculate," button to show a graph of your payout schedule.
- The "Loan Principal" is the remaining amount of the original debt, while the "Interest Rate" is the additional cost of the loan.
- These calculators will show you information about your loan repayment schedule on an amortized basis (credit that is repaid in regular installments), which means you will pay the same amount each month.
- If you pay less than the amount shown, you will have to make a single, very large payment at the end of the loan term, and must pay a larger amount.
Method 2 of 3: Calculating Loan Payments Manually
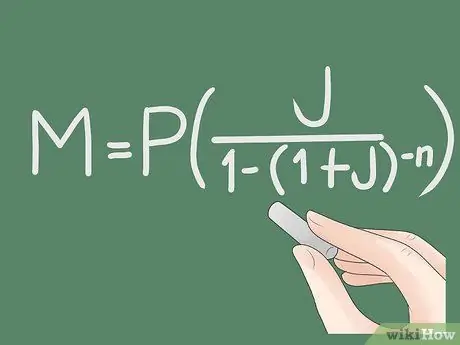
Step 1. Write down the formula used
The formula used to calculate loan payments is M = P * (J / (1 - (1 + J)-N)). Follow these steps to guide you through using this formula, or follow a brief explanation of each variable:
- M = payment amount
- P = principal, meaning the amount of money you borrow
- J = effective interest rate. Bear in mind that this is generally not an annual interest rate, please see the explanation below.
- N = how many times to pay

Step 2. Be careful in rounding the results
Ideally, use a graphing calculator or calculator software to calculate all the formulas in one line. If you are using a calculator that can only perform step-by-step calculations, or if you want to follow the detailed steps below, round to no less than four significant figures before moving on to the next step. Rounding off to a shorter decimal may result in significant rounding errors in your final answer.
- Even simple calculators usually have an "Ans" button. This button is used to enter the previous answer into the next calculation, the result of which will be more accurate than the calculation below.
- The examples below round after each step, but the final step includes the answer you'll get if you complete the calculation in one line, so you can double-check your work.
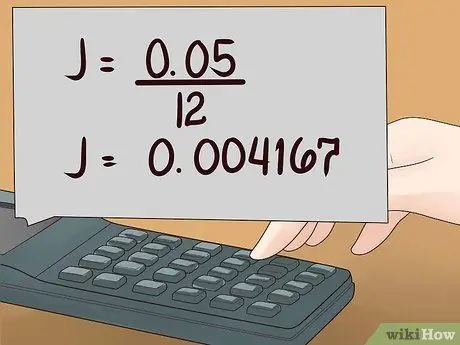
Step 3. Calculate your effective interest rate J
Loan policies will usually state an "annual interest rate," but you may not repay your loan in annual installments. Divide the annual interest rate by 100 to get a decimal, then divide by the number of times you pay your loan each year to get the effective interest rate.
- For example, if your annual interest rate is 5%, and you pay in monthly installments (12 times per year), calculate 5/100 to get 0.05 then calculate J=0.05 / 12 = 0, 004167.
- In unusual cases, interest rates are calculated at different intervals from the payment schedule. Most notably, mortgages in Canada are usually calculated twice per year, although borrowers make payments 12 times per year. In this case, you must divide the annual interest rate by two.

Step 4. Pay attention to the total number of payments N
Your loan policy may already specify this number, or you may have to calculate it yourself. For example, if the term of the loan is 5 years and you will pay in monthly installments 12 times a year, then the total number of your payments is N = 5 * 12 = 60.
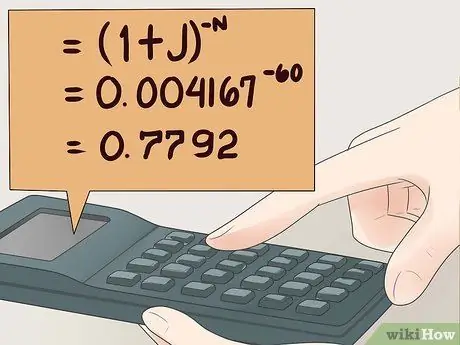
Step 5. Calculate (1+J)-N.
First add 1+J, then increase the answer to the power of "-N." Make sure you put a negative sign in front of N. If your calculator doesn't work with negative exponents, you can write 1/((1+J)N).
In this example, (1+J)-N = (1.004167)-60 = 0, 7792

Step 6. Calculate J/(1-(your answer))
With a simple calculator, first calculate 1 - the number you calculated in the previous step. Then, calculate J divided by the result, using the effective interest rate for the calculation of "J" above.
In this example, J/(1-(your answer)) = 0.004167/(1-0,7792) = 0, 01887

Step 7. Find the amount of your monthly payment
To calculate it, multiply your final result by the loan amount P. The result is the amount of money you have to pay each month to pay off your loan on time.
- For example, if you borrowed $30,000, you would multiply your last answer by 30,000. Continuing the example above, 0.01887 * 30.000 = 566, 1 dollars each month, or $566 and 10 cents.
- This applies to all currencies, not just dollars.
- If you do all these examples in one line using a fancy calculator, you'll get a more accurate main payout answer, coming up very close to $566, 137 or about $566 and 14 cents each month. If we even paid $566 and 10 cents each month as we calculated with the less accurate calculator above, we would have made a slight difference at the end of the loan term, and might have to pay a few dollars more (less than 5 in this case.)
Method 3 of 3: Understanding How Loans Work

Step 1. Understand the difference between a fixed-rate loan and an adjustable-rate loan
All loans use one of these two categories. Make sure you know what type is applied to your loan:
- Loans with fixed interest has a fixed interest rate. Your monthly payment will never change as long as you pay on time.
- Loans with customized flowers will periodically adjust to the current standard interest rate, so you may end up with more or less debt if interest rates change. The interest rate is only recalculated during the "adjustment period" specified in your loan policy. If you know that the current interest rate is only a few months before the next adjustment period, you can plan ahead.

Step 2. Understand amortization
Amortization refers to the rate at which the initial amount you borrowed (the principal loan) is reduced. In general, there are two types of loan repayment schedules:
- Loan payment with full amortization calculated so that you can pay a fixed amount each month over the entire repayment period, paying off the principal and interest rates with each payment. The calculators and formulas above assume that you want a schedule like this.
- Loan repayment plan with just pay the interest giving you a lower initial payment during the "interest-only" period, because you're only paying off the interest, not the initial "principal" loan. After the interest period is over, your monthly payments will be huge, because you will start paying off the principal and interest as well. This will cost you more money in the long run.

Step 3. Pay more money up front to save more money in the long run
Making additional payments will reduce the amount of money you have to spend in the long term, because the smaller the loan amount on which the interest calculation is based. The sooner you do this, the more money you can save.






