There are many different shapes of flat shapes and there are many reasons why you might want to calculate area: from doing homework to estimating how much paint is needed to repaint a living room. Don't worry, wikiHow has the answer! Start from Step 1 below to know how to calculate the area of a plane figure.
Step
Method 1 of 7: Square, Rectangle and Parallelogram
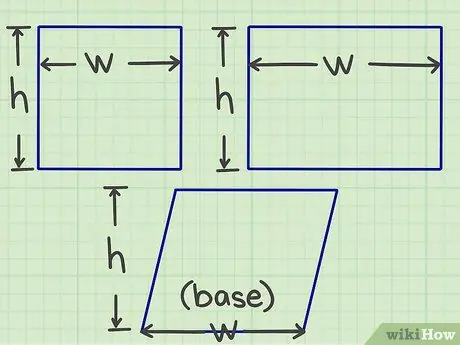
Step 1. Measure the length and width
Start measuring or figuring out the length and width of the flat shape (or in other words, the size of each of the two sides that meet at one point).
- For a parallelogram, you have to find the base and the height, but in simple terms, the idea is the same as length and width.
- In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape.
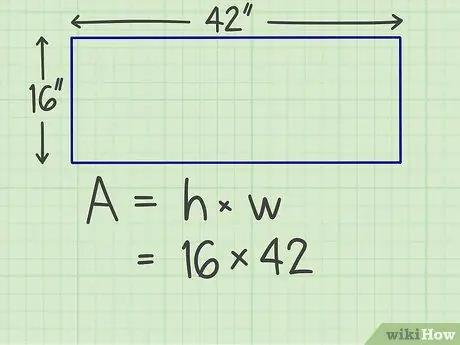
Step 2. Multiply the sides
Multiply one side by the other. For example, suppose you have a rectangle with a width of 16 inches and a length of 42 inches, so you have to calculate 16 x 42.
If you're calculating the area of a square (formerly called a square), save time by squaring one of its sides using a calculator. If the side measures 4 m, press the 4 key and then press the square key on the calculator to display the result. Square means multiplying a number by itself
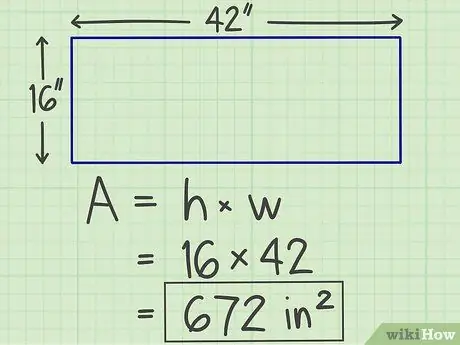
Step 3. Write down the results
The multiplication you did earlier will produce a number, which is the area of the plane you are calculating, with units of "square". Thus, the rectangle we calculated earlier has an area of 672 square inches.
Sometimes this square unit is also written as a small 2 that rises slightly after the unit name (like writing powers)
Method 2 of 7: Trapezoid
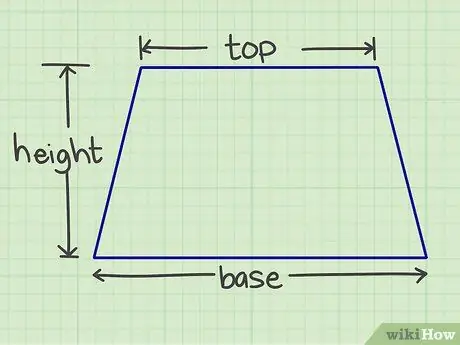
Step 1. Measure the required number
You will need to measure the base, roof and height. The base and the roof are parallel sides, while the height is a perpendicular line connecting the two parallel sides.
In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape
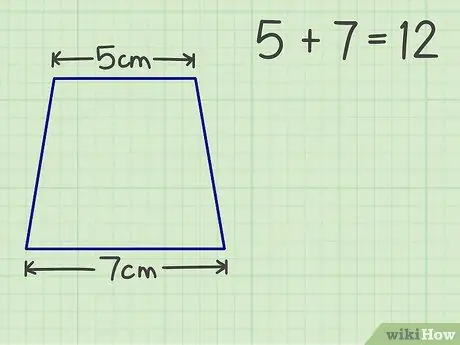
Step 2. Add up the two parallel sides
For example, our trapezoid has a roof of 5 cm and a base of 7 cm. The sum of the two parallel sides is 12.
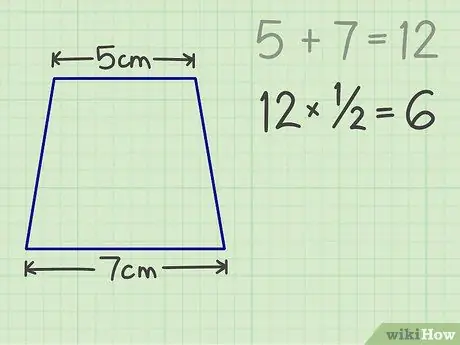
Step 3. Multiply the number by 1/2, the result is 6
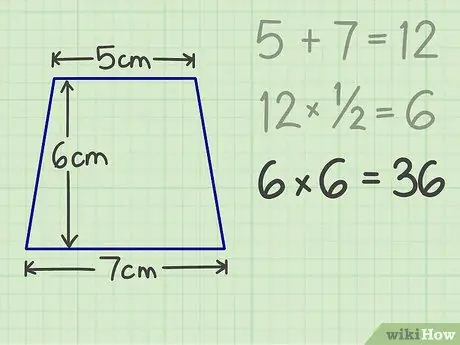
Step 4. After that multiply the result by the height
For example, if a trapezoid has a height of 6 cm, the final result is 36.
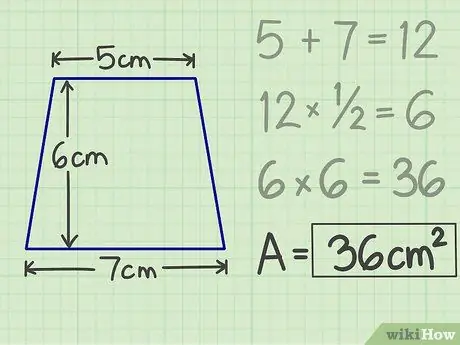
Step 5. Write down the results
The multiplication you did earlier will produce a number, namely the area of the trapezoid. So for a trapezoid with a roof of 5 cm, a base of 7 cm and a height of 6 cm, the area is 36 cm square.
Method 3 of 7: Circle
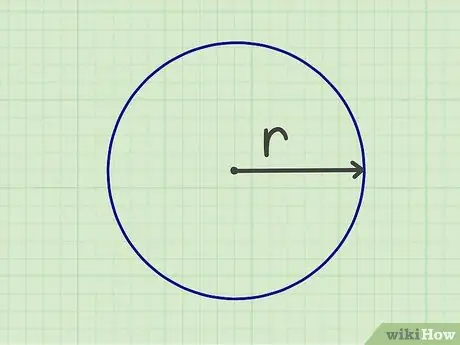
Step 1. Measure the radius
To be able to calculate the area of a circle, you need to measure the radius. the radius is the distance from the center of the circle to its edges. You can also measure the radius by measuring the diameter (the width of the circle from edge to edge), then dividing the number by two.
In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape
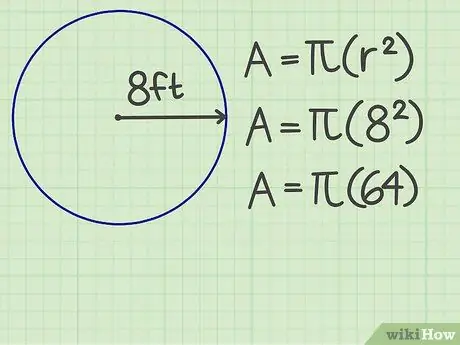
Step 2. Square the fingers
Multiply the number of radii by itself. For example, the radius of a circle is 8 feet, so the result is 64.
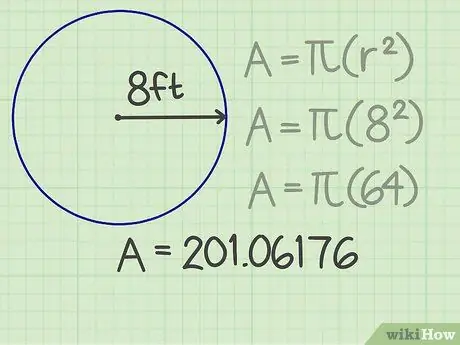
Step 3. Multiply the result by pi
Pi (π) is an important number used in various formulas. If you're using a calculator, press the pi key to get really accurate results. Otherwise, to make your calculations easier, you can round pi to a few digits after the comma, for example, 3, 14159. When you multiply this number by the square of the radius, the result is 201, 06176.
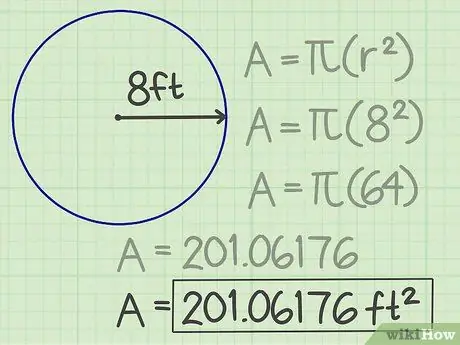
Step 4. Write down the results
The number obtained, 201, 06176 is the area of the circle. Thus, its area is 201,06176 square feet.
Method 4 of 7: Sector (Juring)
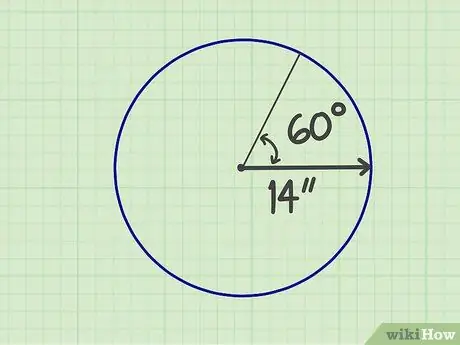
Step 1. Measure the required numbers
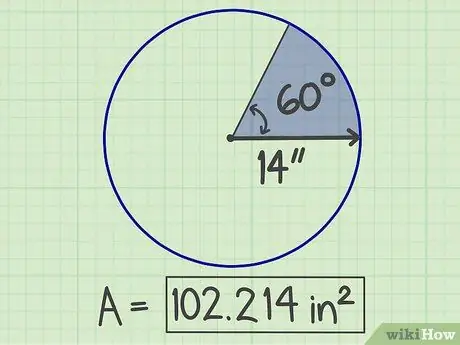
A sector is a section of a circle formed by two radii and an edge, so that the shape is like a fan. You need to know the size of the radius, and the angle that makes up the "fan". For example, suppose a sector has a radius of 14 inches and an angle of 60 degrees.
In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape
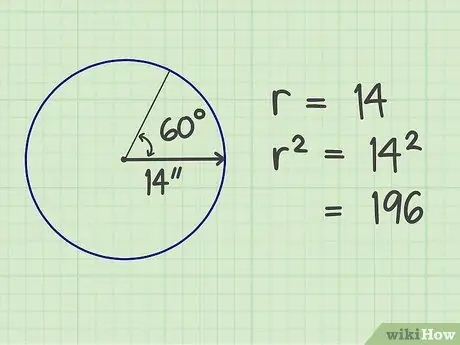
Step 2. Square the fingers
Multiply the number of radii by itself. The result is 196 (14x14).
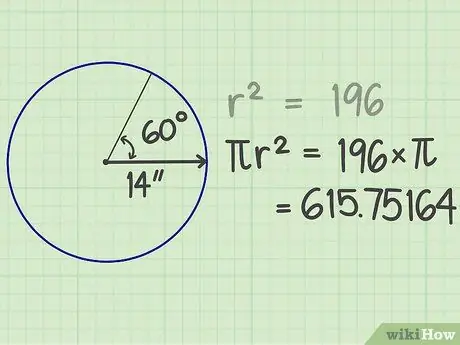
Step 3. Multiply the result by pi
Pi (π) is an important number used in various formulas. If you're using a calculator, press the pi key to get really accurate results. Otherwise, to make your calculations easier, you can round pi to a few digits after the comma, for example 3, 14159. If you multiply this number by the square of the radius, the result is 615, 75164.
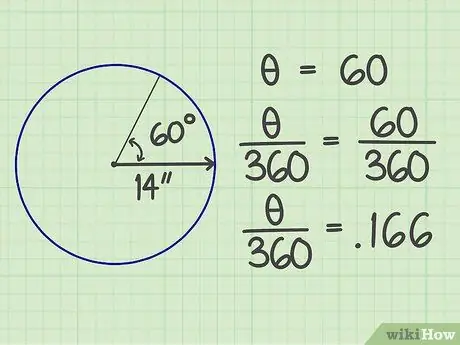
Step 4. Divide the measure of the angle by 360
Divide the sector fan angle by 360 (the measure of a full circle angle). For the example above, the result is around 0.166. If you calculate it with a calculator, the result is actually longer and iterative, but here it is rounded to make the calculation easier.

Step 5. Multiply this number by the previous number
Multiply the number you get after you divide the angle by 360, by the number you get after multiplying pi by the square of the radius. for the example above, the result is 102, 214 (after rounding).
Step 6. Write down the results
The number obtained is the area of the sector, which is 102, 214 square inches.
Method 5 of 7: Ellipse
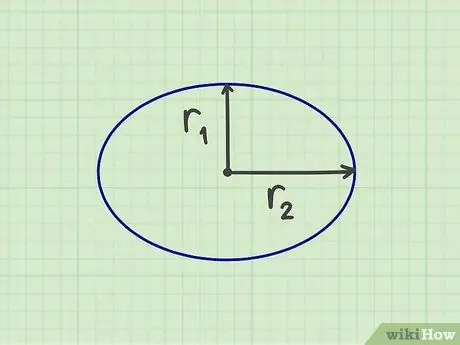
Step 1. Measure the required number
To calculate the area of an ellipse, you need to measure two "radii", namely the short radius and the long radius, which is half the width and half the height of the ellipse. You can also measure the short radius from the center of the ellipse to the shortest side, and the long radius from the center of the ellipse to the longest side. The short radius should form a right angle to the long radius.
In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape
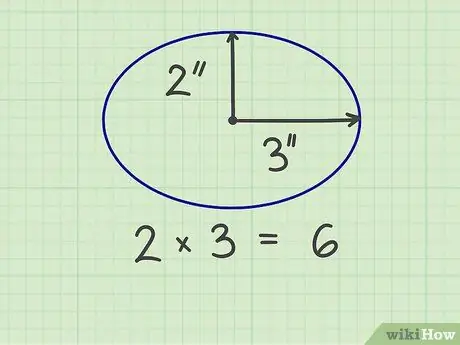
Step 2. Multiply the two fingers
For example, an ellipse is 6 inches wide and 4 inches high, so the radii are 3 inches and 2 inches. When the two numbers are multiplied, the result is 6 (3 x 2).
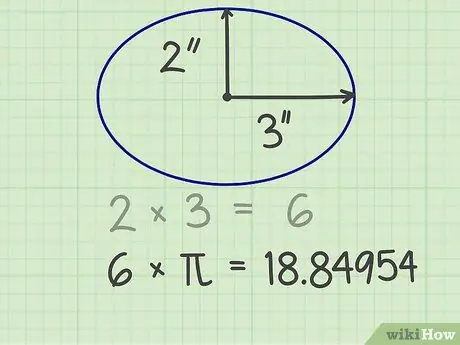
Step 3. Multiply the result by pi
Pi (π) is an important number used in various formulas. If you're using a calculator, press the pi key to get really accurate results. Otherwise, to make your calculations easier, you can round pi to a few digits after the comma, for example, 3, 14159. When you multiply this number by the square of the radius, the result is 18, 84954.

Step 4. Write down the results
The number obtained from the above calculation is the area of the ellipse. In the example above, the area of the ellipse is 18.84954 square inches.
Method 6 of 7: Triangle
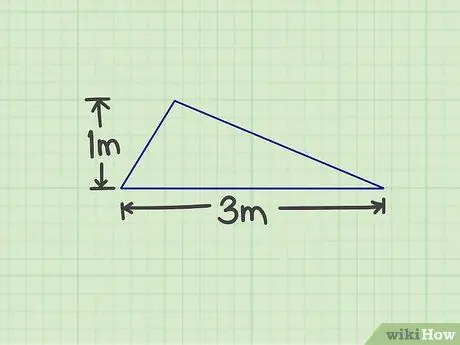
Step 1. Measure the required number
You need to measure the base and height of the triangle. Any side of the triangle can be the base, as long as you can measure the height. For example, there is a triangle with a base of 3 m and a height of 1 m.
In the real world, you may need to measure it yourself, but when it comes to doing homework, the teacher usually has already written down the numbers along with a picture of the shape
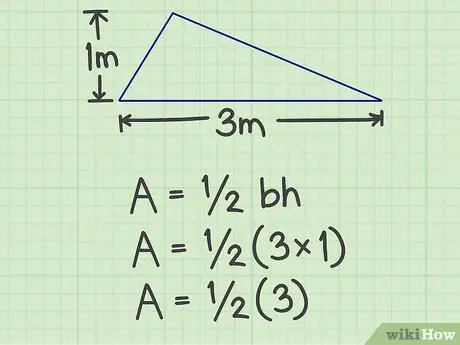
Step 2. Multiply the base by the height
For the example above, the result is 3 (3x1).

Step 3. Multiply the result by 1/2
This multiplication will produce the numbers 1, 5.

Step 4. Write down the results
The number generated by the above calculation is the area of the triangle, and with the example above, its area is 1.5 square meters.
Method 7 of 7: Complex Flat Build
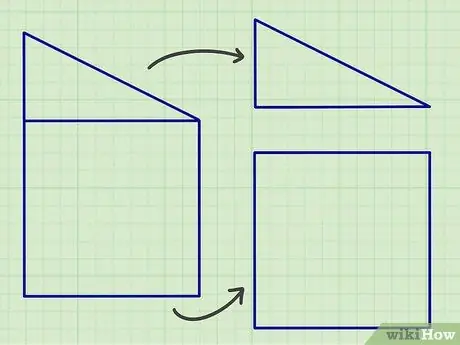
Step 1. Separate complex plane shapes into parts consisting of the standard geometric shapes above
If what you're doing is homework, it might be easy to divide the complex shape into the flat shapes discussed earlier, but in the real world, you might need to divide the flat shape into lots of flat shapes to get an accurate calculation result.
A good way to start is to look for lines that are parallel or that form a certain angle, since most flat shapes are made up of these shapes
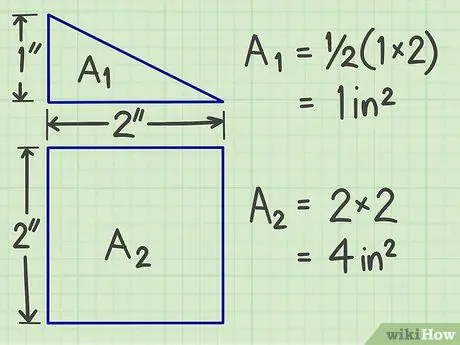
Step 2. Calculate the area for each of the plane figures resulting from the separation
Use the methods above to get the area of each flat shape.
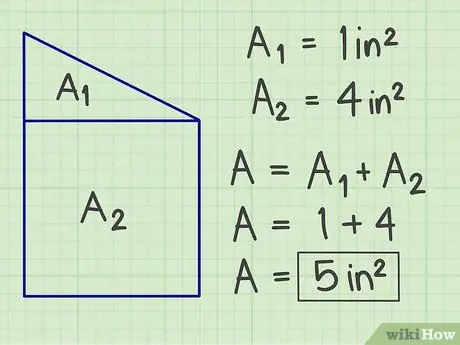
Step 3. Add up the results
Add up all the calculated areas to get the total area of your complex plane figure.
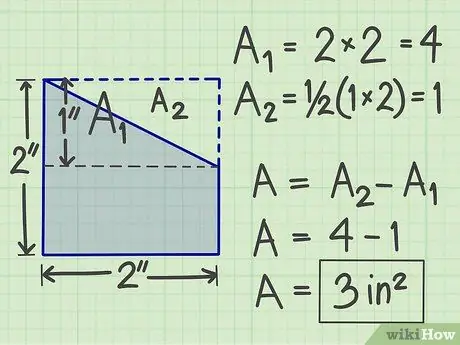
Step 4. Use another method
There are many other ways you can try, depending on the shape of the complex flat shape. For example, you can also add an imaginary plane shape to it so that it becomes a standard geometric plane shape. After that calculate the area, then subtract the area of the imaginary flat shape that you used to add.
Tips
- Use this calculator if you need help solving math calculations.
- Ask a friend for help if you're still having trouble!
Warning
- It's always better to double check the calculation results to make sure they are correct!
- Make sure the units you use are all the same (cm, m, inch, etc.) when calculating, so you don't get miscalculated due to mixed units!






