A rhombus is a quadrilateral with four equal sides. There are three formulas for finding the area of a rhombus. Just follow these steps to know how.
Step
Method 1 of 3: Using diagonal
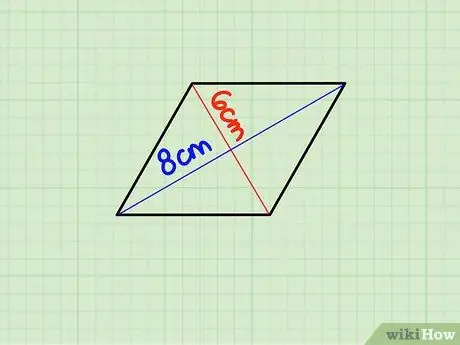
Step 1. Find the length of each diagonal
The diagonals of a rhombus are lines connecting opposite vertices (corners) in the center of the shape. The diagonals of a rhombus are perpendicular and form four right triangles through the point of intersection.
Let's say the diagonal is 6 cm and the length is 8 cm
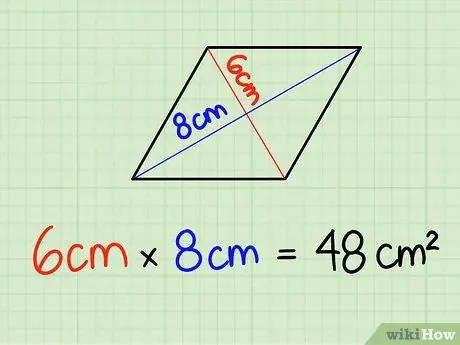
Step 2. Multiply the length of the diagonal
Just write down the length of the diagonal and multiply. In this case, 6 cm x 8 cm = 48 cm2. Don't forget to multiply units because we are working with square units.
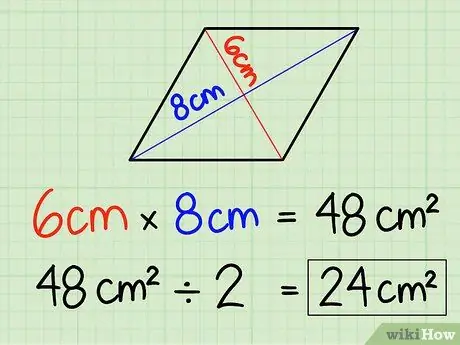
Step 3. Divide the result by 2
Because 6 cm x 8 cm = 48 cm2, just divide the result by 2.48 cm2/2 = 24 cm2. The area of a rhombus is 24 cm2.
Method 2 of 3: Using Base and Height
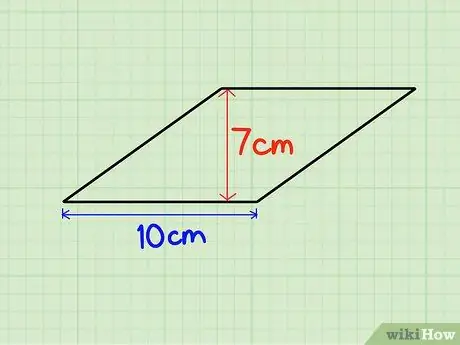
Step 1. Find the base and height
We can also multiply the height of the rhombus by the length of the side of the rhombus. Let's say the height of the rhombus is 7 cm and the base is 10 cm.
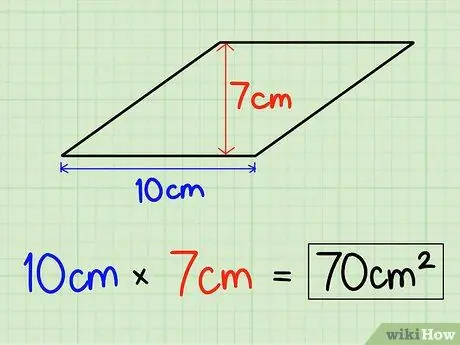
Step 2. Multiply the base and the height
After knowing the base and height of the rhombus, find the area of the shape by multiplying it. So, 10 cm x 7 cm = 70 cm2. The area of a rhombus is 70 cm2.
Method 3 of 3: Using Trigonometry
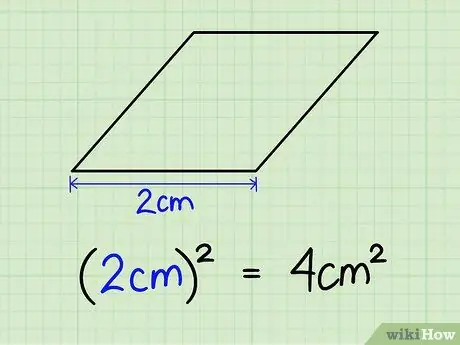
Step 1. Square the length of any side
A rhombus has four equal sides, so it doesn't matter which side we choose. Let's say the side has a length of 2 cm. 2cm x 2cm = 4cm2.
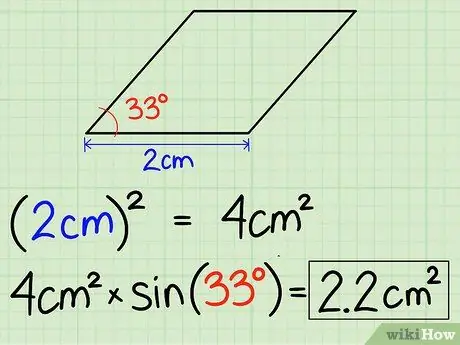
Step 2. Multiply by the sine of one corner
It doesn't matter which angle we choose. Let's say one of the angles is 33 degrees. Just multiply sine (33) by 4 cm2 to get the area of the rhombus. (2cm)2 x sine (33) = 4 cm2 x 1 = 4 cm2. The area of a rhombus is 4 cm2.






