Derivatives can be used to derive useful characteristics from a graph, such as maximum, minimum, peak, trough, and slope values. You can even use it to graph complex equations without a graphing calculator! Unfortunately, working on derivatives is often tedious, but this article will help you with some tips and tricks.
Step

Step 1. Understand derived notation
The following two notations are the most commonly used, although many others can be found here on Wikipedia.
- Leibniz Notation This notation is the most commonly used notation when the equation involves y and x. dy/dx literally means the derivative of y with respect to x. It might be useful to think of it as y/Δx for very different values of x and y. This explanation leads to the definition of the derived limit: limh->0 (f(x+h)-f(x))/h. When using this notation for the second derivative, you should write: d2y/dx2.
- Lagrange Notation The derivative of the function f is also written as f'(x). This notation reads f accented x. This notation is shorter than Leibniz's notation, and is helpful when viewing derivatives as functions. To form a larger degree of derivative, just add ' to f, so the second derivative will be f''(x).

Step 2. Understand the meaning of the derivative and the reason for the descent
First, to find the slope of a linear graph, two points on the line are taken, and their coordinates are entered into the equation (y2 - y1)/(x2 - x1). However, it can only be used for linear graphs. For quadratic equations and higher, the line will be a curve, so finding the difference between two points is not very accurate. To find the slope of the tangent in a curve graph, two points are taken, and put into the general equation to find the slope of the curve graph: [f(x + dx) - f(x)]/dx. Dx denotes delta x, which is the difference between two x coordinates at two points of the graph. Note that this equation is the same as (y2 - y1)/(x2 - x1), only in a different form. Since it was known that the results would be imprecise, an indirect approach was applied. To find the slope of the tangent on (x, f(x)), dx must be close to 0, so that the two drawn points merge into one point. However, you can't divide 0, so once you've entered the two-point values, you'll have to use factoring and other methods to remove dx from the bottom of the equation. Once you've done that, make dx 0 and you're done. This is the slope of the tangent on (x, f(x)). The derivative of an equation is the general equation for finding the slope of any tangent on a graph. This may seem very complicated, but there are some examples below, which will help explain how to get the derivative.
Method 1 of 4: Explicit Derivatives

Step 1. Use an explicit derivative if your equation already has y on one side

Step 2. Plug the equation into the equation [f(x + dx) - f(x)]/dx
For example, if the equation is y = x2, the derivative will be [(x + dx)2 - x2]/dx.
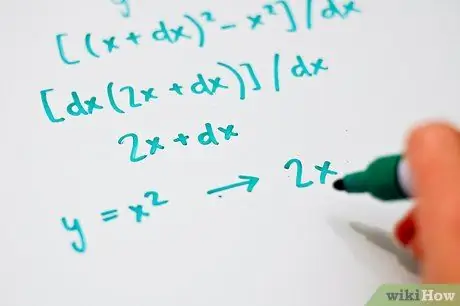
Step 3. Spread and remove dx to form the equation [dx(2x + dx)]/dx
Now, you can throw two dx on the top and bottom. The result is 2x + dx, and as dx approaches zero, the derivative is 2x. This means that the slope of any tangent of the graph y = x2 is 2x. Just enter the x-value for the point for which you want to find the slope.

Step 4. Learn patterns for deriving similar equations
Here are some examples.
- Any exponent is the power times the value, raised to the power less than 1. For example, the derivative of x5 is 5x4, and the derivative of x3, 5 iis3, 5x2, 5. If there is already a number in front of x, just multiply it by the power. For example the derivative of 3x4 is 12x3.
- The derivative of any constant is zero. So, the derivative of 8 is 0.
- The derivative of the sum is the sum of the respective derivatives. For example, the derivative of x3 + 3x2 is 3x2 + 6x.
- The derivative of the product is the first factor times the derivative of the second factor plus the second factor times the derivative of the first factor. For example, the derivative of x3(2x + 1) is x3(2) + (2x + 1)3x2, which is equal to 8x3 + 3x2.
- The derivative of the quotient (say, f/g) is [g(derivative of f) - f(derivative of g)]/g2. For example, the derivative of (x2 + 2x - 21)/(x - 3) is (x2 - 6x + 15)/(x - 3)2.
Method 2 of 4: Implicit Derivatives

Step 1. Use implicit derivatives if your equation cannot already be written with y on one side
In fact, if you wrote y on one side, calculating dy/dx would be tedious. Here's an example of how you can solve this type of equation.
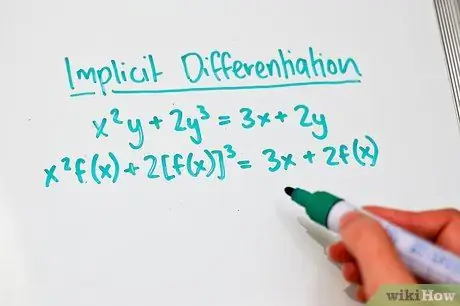
Step 2. In this example, x2y + 2y3 = 3x + 2y, replace y with f(x), so you'll remember that y is actually a function.
The equation then becomes x2f(x) + 2[f(x)]3 = 3x + 2f(x).
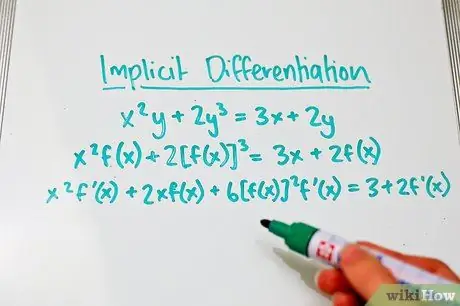
Step 3. To find the derivative of this equation, derive both sides of the equation with respect to x
The equation then becomes x2f'(x) + 2xf(x) + 6[f(x)]2f'(x) = 3 + 2f'(x).
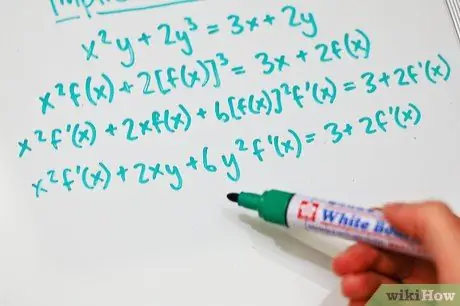
Step 4. Replace f(x) with y again
Be careful not to substitute f'(x), which is different from f(x).
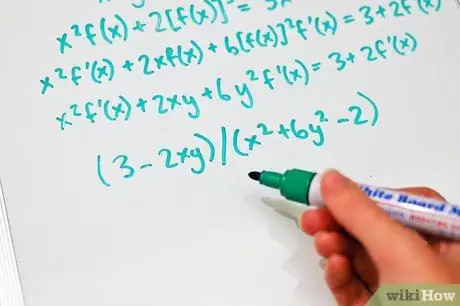
Step 5. Find f'(x)
The answer for this example becomes (3 - 2xy)/(x2 + 6y2 - 2).
Method 3 of 4: Higher Order Derivatives

Step 1. Deriving a function of higher order means that you are deriving the derivative (to order 2)
For example, if the problem asks you to derive third order, then just take the derivative of the derivative of the derivative. For some equations, the higher-order derivative will be 0.
Method 4 of 4: Chain Rule

Step 1. If y is a differential function of z, and z is a differential function of x, y is a composite function of x, and the derivative of y with respect to x (dy/dx) is (dy/du)*(du/dx)
The chain rule can also be a combination of power equations, like this: (2x4 - x)3. To find the derivative, just think of it like the multiplication rule. Multiply the equation by the power and decrease by 1 to the power. Then, multiply the equation by the derivative of the equation in parentheses that raises the power (in this case, 2x^4 - x). The answer to this question is 3(2x4 - x)2(8x3 - 1).
Tips
- Whenever you see a difficult problem to solve, don't worry. Just try to break it down into as many smaller parts as possible by applying the rules of multiplication, quotient, etc. Then, lower each piece.
- Practice with the multiplication rule, the quotient rule, the chain rule, and especially, implicit derivatives, because these rules are much more difficult in calculus.
- Understand your calculator well; try the different functions in your calculator to learn how to use them. It is very useful to know how to use tangents and derivative functions in your calculator if they are available.
- Remember the basic trigonometric derivatives and how to use them.






